Dr. Rogelio Ortigosa
Associate Professor, Applied Mathematics
RESEARCH LINKS
- OrcID: orcid.org/0000-0002-4542-2237
- Scopus Author ID: 56347705900
- MathScinet: 1091151
- ETSII Office B023. Campus Muralla del Mar. Hospital de Marina. C/ Dr. Fleming SN. 30202 Cartagena
- Phone: +34 ---
- Email: rogelio.ortigosa@upct.es
- Available For Postgraduate Supervision
ABOUT
AREAS OF EXPERTISE
PUBLICATIONS
Poya, Roman; Ortigosa, Rogelio; Gil, Antonio J.; Kim, Theodore; Bonet, Javier Generalised tangent stabilised nonlinear elasticity: A powerful framework for controlling material and geometric instabilities Journal Article In: International Journal for Numerical Methods in Engineering, vol. 436, 2025. Ortigosa, Rogelio; Martínez-Frutos, Jesús; Pérez-Escolar, Alberto; Castañar, Inocencio; Ellmer, Nathan; Gil, Antonio J. A generalized theory for physics-augmented neural networks in finite strain thermo-electro-mechanics Journal Article In: Computer Methods in Applied Mechanics and Engineering, vol. 437, pp. 117741, 2025, ISSN: 0045-7825. Gómez-Silva, Francisco; Zaera, Ramon; Ortigosa, Rogelio; Martinez-Frutos, Jesus Topology optimization of lattice structures for target band gaps with optimum volume fraction via Bloch-Floquet theory Journal Article In: Computers & Structures, vol. 307, pp. 107601, 2025, ISSN: 0045-7949. Ellmer, Nathan; Ortigosa, Rogelio; Martinez-Frutos, Jesus; Gil, Antonio J.; Poya, Roman Stretch-based hyperelastic constitutive emulators through Gradient Enhanced Kriging Journal Article In: Computer Methods in Applied Mechanics and Engineering, vol. 423, 2024. Ortigosa, Rogelio; Martinez-Frutos, Jesus; Periago, Francisco Probability-of-failure-based optimization for Random pdes through concentration-of-measure Inequalities Journal Article In: ESAIM: Control, Optimisation and Calculus of Variations, vol. 30, no. 66, 2024. Ortigosa, Rogelio; Martínez-Frutos, Jesús; Mora-Corral, Carlos; Pedregal, Pablo; Periago, Francisco Shape-programming in hyperelasticity through differential growth Journal Article In: Applied Mathematics and Optimization, vol. 89, no. 49, 2024, ISSN: 1432-0606. Klein, Dominik; Ortigosa, Rogelio; Martínez-Frutos, Jesús; Weeger, Oliver Neural networks meet hyperelasticity: On limits of polyconvexity Journal Article Forthcoming In: Journal of the Mechanics and Physics of Solids, Forthcoming. Klein, Dominik; Ortigosa, Rogelio; Martínez-Frutos, Jesús; Weeger, Oliver Nonlinear electro-elastic finite element analysis with neural network constitutive models Journal Article In: Computer Methods in Applied Mechanics and Engineering, vol. 425, 2024, ISBN: 1879-2138. Pérez-Escolar, Alberto; Martinez-Frutos, Jesus; Ortigosa, Rogelio; Ellmer, Nathan; Gil, Antonio J. Learning nonlinear constitutive models in finite strain electromechanics with Gaussian process predictors Journal Article In: Computational Mechanics, 2024, ISBN: 1432-0924. Ellmer, Nathan; Ortigosa, Rogelio; Martinez-Frutos, Jesus; Gil, Antonio J. Gradient enhanced gaussian process regression for constitutive modelling in finite strain hyperelasticity Journal Article In: Computer Methods in Applied Mechanics and Engineering, vol. 418, iss. PART B, pp. 116547, 2024, ISBN: 1879-2138. Poya, Roman; Ortigosa, Rogelio; Gil, Antonio J. Variational schemes and mixed finite elements for large strain isotropic elasticity in principal stretches: Closed‐form tangent eigensystems, convexity conditions, and stabilised elasticity Journal Article In: Numerical Meth Engineering, vol. 124, no. 16, pp. 3436–3493, 2023, ISSN: 1097-0207. Ortigosa, Rogelio; Martínez-Frutos, Jesús; Mora-Corral, Carlos; Pedregal, Pablo; Periago, Francisco Mathematical modeling, analysis and control in soft robotics: a survey Journal Article In: SeMA, 2023, ISSN: 2281-7875. Poya, Roman; Ortigosa, Rogelio; Kim, Theodore Geometric Optimisation Via Spectral Shifting Journal Article In: ACM Trans. Graph., vol. 42, no. 3, pp. 1–15, 2023, ISSN: 1557-7368. Franke, M.; Zähringer, Felix; Hille, Moritz; Ortigosa, Rogelio; Betsch, P.; Gil, Antonio J. A novel mixed and energy‐momentum consistent framework for coupled nonlinear thermo‐electro‐elastodynamics Journal Article In: Numerical Meth Engineering, vol. 124, no. 10, pp. 2135–2170, 2023, ISSN: 1097-0207. Horák, Martin; Gil, Antonio J.; Ortigosa, Rogelio; Kružík, Martin A polyconvex transversely-isotropic invariant-based formulation for electro-mechanics: Stability, minimisers and computational implementation Journal Article In: Computer Methods in Applied Mechanics and Engineering, vol. 403, pp. 115695, 2023, ISSN: 0045-7825. Ortigosa, Rogelio; Martínez-Frutos, Jesús; Gil, Antonio J. Programming shape-morphing electroactive polymers through multi-material topology optimisation Journal Article In: Applied Mathematical Modelling, vol. 118, pp. 346-369, 2023, ISSN: 0307-904X. Remigio-Reyes, Joel Omar; Garduño, Isaías E.; Rojas-García, José Manuel; Arcos-Gutiérrez, Hugo; Ortigosa, Rogelio Topology optimization-driven design of added rib architecture system for enhanced free vibration response of thin-wall plastic components used in the automotive industry Journal Article In: Int J Adv Manuf Technol, vol. 123, no. 3-4, pp. 1231–1247, 2022, ISSN: 1433-3015. BibTeX | Links: Ortigosa, Rogelio; Martínez-Frutos, Jesús; Gil, Antonio J. A computational framework for topology optimisation of flexoelectricity at finite strains considering a multi-field micromorphic approach Journal Article In: Computer Methods in Applied Mechanics and Engineering, vol. 401, pp. 115604, 2022, ISSN: 0045-7825. Franke, M.; Ortigosa, Rogelio; Martínez-Frutos, Jesús; Gil, Antonio J.; Betsch, P. A thermodynamically consistent time integration scheme for non-linear thermo-electro-mechanics Journal Article In: Computer Methods in Applied Mechanics and Engineering, vol. 389, pp. 114298, 2022, ISSN: 0045-7825. Marín, Francisco J.; Ortigosa, Rogelio; Martínez-Frutos, Jesús; Gil, Antonio J. Viscoelastic up-scaling rank-one effects in in-silico modelling of electro-active polymers Journal Article In: Computer Methods in Applied Mechanics and Engineering, vol. 389, pp. 114358, 2022, ISSN: 0045-7825. Ortigosa, Rogelio; Martínez-Frutos, Jesús; Mora-Corral, Carlos; Pedregal, Pablo; Periago, Francisco Optimal control and design of magnetic field-responsive smart polymer composites Journal Article In: Applied Mathematical Modelling, vol. 103, pp. 141-161, 2022, ISSN: 0307-904X. Klein, Dominik K.; Ortigosa, Rogelio; Martínez-Frutos, Jesús; Weeger, Oliver Finite electro-elasticity with physics-augmented neural networks Journal Article In: Computer Methods in Applied Mechanics and Engineering, vol. 400, pp. 115501, 2022, ISSN: 0045-7825. Ortigosa, Rogelio; Martínez-Frutos, Jesús Topology optimisation of stiffeners layout for shape-morphing of dielectric elastomers Journal Article In: Struct Multidisc Optim, vol. 64, no. 6, pp. 3681–3703, 2021, ISSN: 1615-1488. BibTeX | Links: Martínez-Frutos, Jesús; Ortigosa, Rogelio Risk-averse approach for topology optimization of fail-safe structures using the level-set method Journal Article In: Comput Mech, vol. 68, no. 5, pp. 1039–1061, 2021, ISSN: 1432-0924. BibTeX | Links: Ortigosa, Rogelio; Martínez-Frutos, Jesús Multi-resolution methods for the topology optimization of nonlinear electro-active polymers at large strains Journal Article In: Comput Mech, vol. 68, no. 2, pp. 271–293, 2021, ISSN: 1432-0924. BibTeX | Links: Ortigosa, Rogelio; Martínez-Frutos, Jesús; Ruiz, David; Donoso, Alberto; Bellido, Jose C. Density-based topology optimisation considering nonlinear electromechanics Journal Article In: Struct Multidisc Optim, vol. 64, no. 1, pp. 257–280, 2021, ISSN: 1615-1488. BibTeX | Links: Martínez-Frutos, Jesús; Ortigosa, Rogelio Robust topology optimization of continuum structures under uncertain partial collapses Journal Article In: Computers & Structures, vol. 257, pp. 106677, 2021, ISSN: 0045-7949. Marín, Francisco J.; Martínez-Frutos, Jesús; Ortigosa, Rogelio; Gil, Antonio J. A Convex Multi-Variable based computational framework for multilayered electro-active polymers Journal Article In: Computer Methods in Applied Mechanics and Engineering, vol. 374, pp. 113567, 2021, ISSN: 0045-7825. Martínez-Frutos, Jesús; Ortigosa, Rogelio Robust topology optimization of continuum structures under uncertain partial collapses Journal Article In: Computers & Structures, vol. 257, pp. 106677, 2021, ISSN: 0045-7949. Ortigosa, Rogelio; Martínez-Frutos, Jesús; Mora-Corral, Carlos; Pedregal, Pablo; Periago, Francisco Optimal Control of Soft Materials Using a Hausdorff Distance Functional Journal Article In: SIAM Journal on Control and Optimization, vol. 59, no. 1, pp. 393-416, 2021. Martínez-Frutos, Jesús; Ortigosa, Rogelio; Gil, Antonio J. In-silico design of electrode meso-architecture for shape morphing dielectric elastomers Journal Article In: Journal of the Mechanics and Physics of Solids, vol. 157, pp. 104594, 2021, ISSN: 0022-5096. Ortigosa, Rogelio; Gil, Antonio J.; Martínez-Frutos, Jesus; Franke, M.; Bonet, Javier A new energy–momentum time integration scheme for non-linear thermo-mechanics Journal Article In: Computer Methods in Applied Mechanics and Engineering, vol. 372, pp. 113395, 2020, ISSN: 0045-7825. Martínez-Frutos, Jesus; Ortigosa, Rogelio; Pedregal, Pablo; Periago, Francisco Robust optimal control of stochastic hyperelastic materials Journal Article In: Applied Mathematical Modelling, vol. 88, pp. 888-904, 2020, ISSN: 0307-904X. Ortigosa, Rogelio; Martínez-Frutos, Jesús; Gil, Antonio J. A new stabilisation approach for level-set based topology optimisation of hyperelastic materials Journal Article In: Struct Multidisc Optim, vol. 60, pp. 2343–2371, 2019, ISBN: 1615-147X. Ortigosa, Rogelio; Franke, M.; Janz, A.; Gil, Antonio J.; Betsch, P. An energy-momentum integration scheme based on a convex multi-variable framework for non-linear electro-elastodynamics Journal Article In: Computer Methods in Applied Mechanics and Engineering, vol. 339, pp. 1-35, 2018, ISSN: 0045-7825. Garcia-Blanco, Emilio; Ortigosa, Rogelio; Gil, Antonio J.; Lee, C. H.; Bonet, Javier A polyconvex computational formulation for electro-activation in cardiac mechanics Journal Article In: Biomechanics and Modeling in Mechanobiology, 2018. Poya, Roman; Gil, Antonio J.; Ortigosa, Rogelio; Palma, Roberto On a family of numerical models for couple stress based exoelectricity for continua and beams Journal Article In: Journal of the Mechanics and Physics of Solids, 2018. Poya, Roman; Gil, Antonio J.; Ortigosa, Rogelio A curvilinear high order finite element framework for electro-mechanics: From linearised electro-elasticity to massively deformable dielectric elastomers Journal Article In: Computer Methods in Applied Mechanics and Engineering, vol. 329, pp. 75-117, 2018. Poya, Roman; Ortigosa, Rogelio; Gil, Antonio J. A high performance data parallel tensor contraction framework: Application to coupled electro-mechanics Journal Article In: Computer Physics Communications, vol. 216, pp. 35-52, 2017, ISSN: 0010-4655. Hesch, C.; Gil, Antonio J.; Ortigosa, Rogelio; Dittmann, M.; Bilgen, C.; Betsch, P.; Franke, M.; Janz, A.; K.Weinberg, A framework for polyconvex large strain phase-field methods to fracture Journal Article In: Computer Methods in Applied Mechanics and Engineering, vol. 317, pp. 649-683, 2017, ISSN: 0045-7825. Ortigosa, Rogelio; Gil, Antonio J.; Lee, C. H. A computational framework for large strain nearly and truly incompressible electromechanics based on convex multi-variable strain energies Journal Article In: Computer Methods in Applied Mechanics and Engineering, vol. 310, pp. 297-334, 2016, ISSN: 0045-7825. Ortigosa, Rogelio; Gil, Antonio J. A new framework for large strain electromechanics based on convex multi-variable strain energies: Conservation laws, hyperbolicity and extension to electro-magneto- mechanics Journal Article In: Computer Methods in Applied Mechanics and Engineering,, vol. 309, pp. 202-242, 2016, ISSN: 0045-7825. Bonet, Javier; Gil, Antonio J.; Ortigosa, Rogelio On a tensor cross product based formulation of large strain solid mechanics Journal Article In: International Journal of Solids and Structures, vol. 84, pp. 49-63, 2016, ISSN: 0020-7683. Ortigosa, Rogelio; Gil, Antonio J. A new framework for large strain electromechanics based on convex multi- variable strain energies: Finite Element discretisation and computational implementation Journal Article In: Computer Methods in Applied Mechanics and Engineering, vol. 302, pp. 329-360, 2016, ISSN: 0045-7825. Ortigosa, Rogelio; Gil, Antonio J. A new framework for large strain electromechanics based on convex multi- variable strain energies: variational formulation and material characterisation Journal Article In: Computer Methods in Applied Mechanics and Engineering, vol. 302, pp. 293-328, 2016. Gil, Antonio J.; Lee, C. H.; Bonet, Javier; Ortigosa, Rogelio A first order hyperbolic framework for large strain computational solid dynamics. Part II: Total Lagrangian compressible, nearly incompressible and truly incompressible elasticity Journal Article In: Computer Methods in Applied Mechanics and Engineering, vol. 300, pp. 146-181, 2016, ISSN: 0045-7825. Ortigosa, Rogelio; Gil, Antonio J.; Bonet, Javier; Hesch, Christian A computational framework for polyconvex large strain elasticity for geometrically exact beam theory Journal Article In: Computational Mechanics, vol. 57, pp. 277–303, 2016, ISSN: 0178-7675.2025

@article{Poya2024,
title = {Generalised tangent stabilised nonlinear elasticity: A powerful framework for controlling material and geometric instabilities},
author = {Roman Poya and Rogelio Ortigosa and Antonio J. Gil and Theodore Kim and Javier Bonet},
doi = {https://doi.org/10.1016/j.cma.2024.117701},
year = {2025},
date = {2025-03-01},
urldate = {2025-03-01},
journal = {International Journal for Numerical Methods in Engineering},
volume = {436},
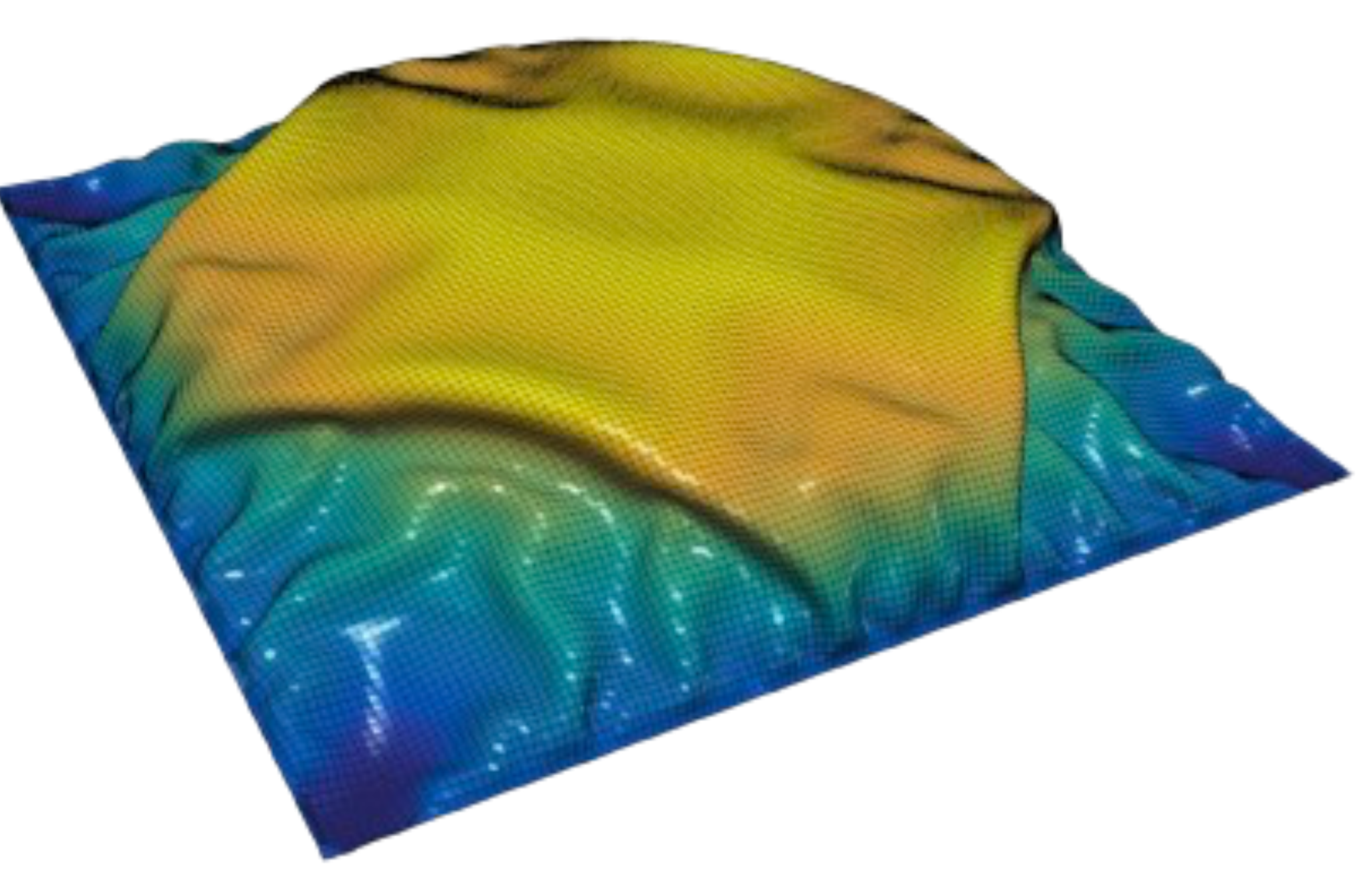
abstract = {Tangent stabilised large strain isotropic elasticity was recently proposed by Poya et al. (2023) wherein by working directly with principal stretches the entire eigenstructure of constitutive and geometric/initial stiffness terms were found in closed-form, giving fresh insights into exact convexity conditions of highly non-convex functions in discrete settings. Consequently, owing to these newly found tangent eigenvalues an analytic tangent stabilisation was proposed (for common non-convex strain energies that exhibit material and/or geometric instabilities) bypassing incumbent numerical approaches routinely used in nonlinear finite element analysis. This formulation appears to be extremely robust for quasi-static simulation of complex deformations even with no load increments and time stepping while still capturing instabilities (similar to dynamic analysis) automatically in ways that are infeasible for path-following techniques in practice. In this work, we generalise the notion of tangent stabilised elasticity to virtually all known invariant formulations of nonlinear elasticity. We show that, closed-form eigen-decomposition of tangents is easily available irrespective of invariant formulation or integrity basis. In particular, we work out closed-form tangent eigensystems for isotropic Total Lagrangian deformation gradient ()-based and right Cauchy–Green ()-based as well as Updated Lagrangian left Cauchy–Green ()-based formulations and present their exact convexity conditions postulated in terms of their corresponding tangent and geometric stiffness eigenvalues. In addition, we introduce the notion of geometrically stabilised polyconvex large strain elasticity for models that are materially stable but exhibit geometric instabilities for whom we construct their geometric stiffness in a spectrally-decomposed form analytically. We further extend this framework to the case of transverse isotropy where once again, closed-form tangent eigensystems are found for common transversely isotropic invariants. In this context, we augment the recent work on mixed variational formulations in principal stretches for deformable and rigid bodies, by presenting a mixed variational formulation for models with arbitrarily directed inextensible fibres. Since, tangent stabilisation unleashes an unparallelled capability for extreme deformations new numerical techniques are required to guarantee element-inversion-safe analysis. To this end, we propose a discretisation-aware load-stepping together with a line search scheme for a robust industry-grade implementation of tangent stabilised elasticity over general polyhedral meshes. Extensive comparisons with path-following techniques provide conclusive evidence that utilising tangent stabilised elasticity can offer both faster and automated results.},
keywords = {},
pubstate = {published},
tppubtype = {article}
}

@article{ORTIGOSA2025117741,
title = {A generalized theory for physics-augmented neural networks in finite strain thermo-electro-mechanics},
author = {Rogelio Ortigosa and Jesús Martínez-Frutos and Alberto Pérez-Escolar and Inocencio Castañar and Nathan Ellmer and Antonio J. Gil},
url = {https://www.sciencedirect.com/science/article/pii/S0045782525000131},
doi = {https://doi.org/10.1016/j.cma.2025.117741},
issn = {0045-7825},
year = {2025},
date = {2025-01-01},
urldate = {2025-01-01},
journal = {Computer Methods in Applied Mechanics and Engineering},
volume = {437},
pages = {117741},
abstract = {This manuscript introduces a novel neural network-based computational framework for constitutive modeling of thermo-electro-mechanically coupled materials at finite strains, with four key innovations: (i) It supports calibration of neural network models with various input forms, such as Ψnn(F,E0,θ), enn(F,D0,η), Υnn(F,E0,η), or Γnn(F,D0,θ), with F representing the deformation gradient tensor, E0 and D0 the electric field and electric displacement field, respectively and finally, θ and η, the temperature and entropy fields. These models comply with physical laws and material symmetries by utilizing isotropic or anisotropic invariants corresponding to the material’s symmetry group. (ii) A calibration approach is developed for the case of experimental data, where entropy η is typically unmeasurable. (iii) The framework accommodates models like enn(F,D0,η), specially convenient for the imposition of polyconvexity across the three physics involved. A detailed calibration study is conducted evaluating various neural network architectures and considering a large variety of ground truth thermo-electro-mechanical constitutive models. The results demonstrate excellent predictive performance on larger datasets, validated through complex finite element simulations using both ground truth and neural network-based models. Crucially, the framework can be straightforwardly extended to scenarios involving other physics.},
keywords = {},
pubstate = {published},
tppubtype = {article}
}

@article{GOMEZSILVA2025107601,
title = {Topology optimization of lattice structures for target band gaps with optimum volume fraction via Bloch-Floquet theory},
author = {Francisco Gómez-Silva and Ramon Zaera and Rogelio Ortigosa and Jesus Martinez-Frutos},
url = {https://www.sciencedirect.com/science/article/pii/S0045794924003304},
doi = {https://doi.org/10.1016/j.compstruc.2024.107601},
issn = {0045-7949},
year = {2025},
date = {2025-01-01},
urldate = {2025-01-01},
journal = {Computers & Structures},
volume = {307},
pages = {107601},
abstract = {In this work, a topology optimization algorithm has been developed to design bi-material lattice structures showing a band gap around a target frequency, using just one unit cell through the application of Bloch-Floquet theorem. The Bidirectional Evolutionary Structural optimization (BESO) method has been employed, based on bi-material interpolation. A new objective function has been defined, which uses only the natural frequencies closest to the target one, regardless of their position with respect to the fundamental natural frequency. This reduces the computational cost by limiting the number of frequencies considered, and improves the robustness of the optimization process, as these frequencies adapt to changes in the distribution of materials within the domain, constantly encompassing the target frequency. In addition, a novel approach has been implemented to determine the optimal volume fraction of the materials forming the structure, a parameter typically predefined in other works before starting the optimization process. Consequently, the algorithm can autonomously identify the volume that produces the widest band gap around the target frequency. The algorithm has been evaluated for different cases of lattice structures formed by the periodic repetition of a unit cell in both 1D (1D-CR) and 2D (2D-CR), comparing some results with those obtained in other works through different approaches.},
keywords = {},
pubstate = {published},
tppubtype = {article}
}
2024

@article{Ellmer0000,
title = {Stretch-based hyperelastic constitutive emulators through Gradient Enhanced Kriging},
author = {Nathan Ellmer and Rogelio Ortigosa and Jesus Martinez-Frutos and Antonio J. Gil and Roman Poya},
doi = {https://doi.org/10.1016/j.cma.2024.117408},
year = {2024},
date = {2024-12-01},
urldate = {2024-12-01},
journal = {Computer Methods in Applied Mechanics and Engineering},
volume = {423},
abstract = {This paper introduces a new Gradient Enhanced Gaussian Predictor (Kriging) constitutive metamodel based on the use of principal stretches for hyperelasticity. The model further accounts for anisotropy by incorporating suitable invariants of the relevant symmetry integrity basis. The use of stretches is beneficial since it aligns to experimental practices for data gathering, removes the challenge associated with stress projections in isotropy, and increases the range of available constitutive models. This paper presents three significant novelties. The first arises from the proposed approach highlighting the need to enforce physical symmetries and resulted in the authors altering the standard Radial Basis style correlation function to incorporate invariants which naturally uphold these symmetries. The invariants used are both the commonly employed invariants of the right Cauchy–Green strain tensor and the lesser used invariants of the stretch tensor. Note that one may consider using invariants in the correlation function to be the same as using invariants for inputs to the metamodel and this would be true if Ordinary Kriging was used. But the derivatives used in the chain rule clearly result in a new formulation. Secondly, the authors compare two approaches to the infill strategies, one consisting of the error in stress and the other utilising uncertainty provided by Kriging directly. This enables Kriging to guide the user as to most efficient data to insert into the dataset. The final novelty involves the integration of calibrated constitutive metamodels into Finite Element simulations thereby showcasing the accuracy yielded even when handling highly complex deformations such as bending, wrinkling and pinching. Furthermore, the constitutive models are calibrated with data from both isotropic and anisotropic materials such as rank-one laminates, making the accuracy achieved with the small calibration sets even more impressive. The formulation is shown to perform equally well for both synthetic and experimental type of collected data.
},
keywords = {},
pubstate = {published},
tppubtype = {article}
}
@article{Ortigosa2024,
title = {Probability-of-failure-based optimization for Random pdes through concentration-of-measure Inequalities},
author = {Rogelio Ortigosa and Jesus Martinez-Frutos and Francisco Periago},
doi = {https://doi.org/10.1051/cocv/2023075},
year = {2024},
date = {2024-09-23},
urldate = {2024-03-01},
journal = {ESAIM: Control, Optimisation and Calculus of Variations},
volume = {30},
number = {66},
abstract = {Control and optimization problems constrained by partial differential equations (PDEs)
with random input data and that incorporate probabilities of failure in their formulations are numerically
extremely challenging, since the computational cost of estimating the tails of a probability
distribution is prohibitive in many situations encountered in real-life engineering problems. In addition,
probabilities of failure are often discontinuous and include huge flat regions where gradients vanish.
Based on the McDiarmid concentration-of-measure inequality, this paper proposes a new functional
which provides a tight and smooth bound for the probability of a given random functional of exceeding
a prescribed threshold parameter. Hence, this approach relieves the above-mentioned difficulties in
the case where the solution map is convex with respect to the random parameter, as in the case of
a deterministic differential operator and the random parameter appearing linearly in the right-hand
side term. Well-posedness of the corresponding optimal control problem is established and the viability
of the proposed method is numerically illustrated by two benchmarks examples arising in topology
optimization and optimal control theory.},
keywords = {},
pubstate = {published},
tppubtype = {article}
}
with random input data and that incorporate probabilities of failure in their formulations are numerically
extremely challenging, since the computational cost of estimating the tails of a probability
distribution is prohibitive in many situations encountered in real-life engineering problems. In addition,
probabilities of failure are often discontinuous and include huge flat regions where gradients vanish.
Based on the McDiarmid concentration-of-measure inequality, this paper proposes a new functional
which provides a tight and smooth bound for the probability of a given random functional of exceeding
a prescribed threshold parameter. Hence, this approach relieves the above-mentioned difficulties in
the case where the solution map is convex with respect to the random parameter, as in the case of
a deterministic differential operator and the random parameter appearing linearly in the right-hand
side term. Well-posedness of the corresponding optimal control problem is established and the viability
of the proposed method is numerically illustrated by two benchmarks examples arising in topology
optimization and optimal control theory.@article{Ortigosa2024b,
title = {Shape-programming in hyperelasticity through differential growth},
author = {Rogelio Ortigosa and Jesús Martínez-Frutos and Carlos Mora-Corral and Pablo Pedregal and Francisco Periago},
editor = {Springer},
url = {https://link.springer.com/10.1007/s00245-024-10117-6?utm_source=rct_congratemailt&utm_medium=email&utm_campaign=oa_20240323&utm_content=10.1007/s00245-024-10117-6},
doi = {10.1007/s00245-024-10117-6},
issn = {1432-0606},
year = {2024},
date = {2024-03-23},
urldate = {2024-12-01},
journal = {Applied Mathematics and Optimization},
volume = {89},
number = {49},
abstract = {This paper is concerned with the growth-driven shape-programming problem, which involves determining a growth tensor that can produce a deformation on a hyperelastic body reaching a given target shape. We consider the two cases of globally compatible growth, where the growth tensor is a deformation gradient over the undeformed domain, and the incompatible one, which discards such hypothesis. We formulate the problem within the framework of optimal control theory in hyperelasticity. The Hausdorff distance is used to quantify dissimilarities between shapes; the complexity of the actuation is incorporated in the cost functional as well. Boundary conditions and external loads are allowed in the state law, thus extending previous works where the stress-free hypothesis turns out to be essential. A rigorous mathematical analysis is then carried out to prove the well-posedness of the problem. The numerical approximation is performed using gradient-based optimisation algorithms. Our main goal in this part is to show the possibility to apply inverse techniques for the numerical approximation of this problem, which allows us to address more generic situations than those covered by analytical approaches. Several numerical experiments for beam-like and shell-type geometries illustrate the performance of the proposed numerical scheme.},
keywords = {},
pubstate = {published},
tppubtype = {article}
}

@article{Klein2024b,
title = {Neural networks meet hyperelasticity: On limits of polyconvexity},
author = {Dominik Klein and Rogelio Ortigosa and Jesús Martínez-Frutos and Oliver Weeger},
editor = {Elsevier},
year = {2024},
date = {2024-03-15},
urldate = {2024-03-15},
journal = {Journal of the Mechanics and Physics of Solids},
keywords = {},
pubstate = {forthcoming},
tppubtype = {article}
}
@article{Klein2024,
title = {Nonlinear electro-elastic finite element analysis with neural network constitutive models},
author = {Dominik Klein and Rogelio Ortigosa and Jesús Martínez-Frutos and Oliver Weeger},
editor = {Elsevier},
url = {https://www.sciencedirect.com/science/article/pii/S004578252400166X},
doi = {https://doi.org/10.1016/j.cma.2024.116910},
isbn = {1879-2138},
year = {2024},
date = {2024-03-15},
urldate = {2024-07-01},
journal = {Computer Methods in Applied Mechanics and Engineering},
volume = {425},
abstract = {In the present work, the applicability of physics-augmented neural network (PANN) constitutive models for complex electro-elastic finite element analysis is demonstrated. For the investigations, PANN models for electro-elastic material behavior at finite deformations are calibrated to different synthetically generated datasets describing the constitutive response of dielectric elastomers. These include an analytical isotropic potential, a homogenised rank-one laminate, and a homogenised metamaterial with a spherical inclusion. Subsequently, boundary value problems inspired by engineering applications of composite electro-elastic materials are considered. Scenarios with large electrically induced deformations and instabilities are particularly challenging and thus necessitate extensive investigations of the PANN constitutive models in the context of finite element analyses. First of all, an excellent prediction quality of the model is required for very general load cases occurring in the simulation. Furthermore, simulation of large deformations and instabilities poses challenges on the stability of the numerical solver, which is closely related to the constitutive model. In all cases studied, the PANN models yield excellent prediction qualities and a stable numerical behavior even in highly nonlinear scenarios. This can be traced back to the PANN models excellent performance in learning both the first and second derivatives of the ground truth electro-elastic potentials, even though it is only calibrated on the first derivatives. Overall, this work demonstrates the applicability of PANN constitutive models for the efficient and robust simulation of engineering applications of composite electro-elastic materials.
},
keywords = {},
pubstate = {published},
tppubtype = {article}
}
@article{Pérez-Escolar2024,
title = {Learning nonlinear constitutive models in finite strain electromechanics with Gaussian process predictors},
author = {Alberto Pérez-Escolar and Jesus Martinez-Frutos and Rogelio Ortigosa and Nathan Ellmer and Antonio J. Gil},
editor = {Springer},
doi = {10.1007/s00466-024-02446-8},
isbn = {1432-0924},
year = {2024},
date = {2024-02-20},
urldate = {2024-03-01},
journal = {Computational Mechanics},
abstract = {This paper introduces a metamodelling technique that employs gradient-enhanced Gaussian Process Regression (GPR) to emulate diverse internal energy densities based on the deformation gradient tensor F and electric displacement eld D0. The approach integrates principal invariants as inputs for the surrogate internal energy density, enforcing physical constraints like material frame indi erence and symmetry. This technique enables accurate interpolation of energy and its derivatives, including the rst Piola-Kirchho stress tensor and material electric field. The method ensures stress and electric eld-free conditions at the origin, which is challenging with regression-based methods like neural networks. The paper highlights that using invariants of the dual potential of internal energy density, i.e., the free energy density dependent on the material electric eld E0, is inappropriate. The saddle point nature of the latter contrasts with the convexity of the internal energy density, creating challenges for GPR or Gradient Enhanced GPR models using invariants of F and E0 (free energy-based GPR), compared to those involving F and D0 (internal energy-based GPR). Numerical examples within a 3D Finite Element framework assess surrogate
model accuracy across challenging scenarios, comparing displacement and stress elds with ground-truth analytical models. Cases include extreme twisting and electrically induced wrinkles, demonstrating practical applicability and robustness of the proposed approach.},
keywords = {},
pubstate = {published},
tppubtype = {article}
}
model accuracy across challenging scenarios, comparing displacement and stress elds with ground-truth analytical models. Cases include extreme twisting and electrically induced wrinkles, demonstrating practical applicability and robustness of the proposed approach.@article{Ellmer2024,
title = {Gradient enhanced gaussian process regression for constitutive modelling in finite strain hyperelasticity},
author = {Nathan Ellmer and Rogelio Ortigosa and Jesus Martinez-Frutos and Antonio J. Gil},
editor = {Elsevier},
doi = {10.1016/j.cma.2023.116547},
isbn = {1879-2138},
year = {2024},
date = {2024-01-05},
urldate = {2024-01-05},
journal = {Computer Methods in Applied Mechanics and Engineering},
volume = {418},
issue = {PART B},
pages = {116547},
abstract = {This paper introduces a metamodelling technique that leverages gradient-enhanced Gaussian process regression (also known as gradient-enhanced Kriging), effectively emulating the response of diverse hyperelastic strain energy densities. The approach adopted incorporates principal invariants as inputs for the surrogate of the strain energy density. This integration enables the surrogate to inherently enforce fundamental physical constraints, such as material frame indifference and material symmetry, right from the outset. The proposed approach provides accurate interpolation for energy and the first Piola–Kirchhoff stress tensor (e.g. first order derivatives with respect to inputs). The paper presents three notable innovations. Firstly, it introduces the utilisation of Gradient-Enhanced Kriging to approximate a diverse range of phenomenological models, encompassing numerous isotropic hyperelastic strain energies and a transversely isotropic potential. Secondly, this study marks the inaugural application of this technique for approximating the effective response of composite materials. This includes rank-one laminates, for which analytical solutions are feasible. However, it also encompasses more complex composite materials characterised by a Representative Volume Element (RVE) comprising an elastomeric matrix with a centred spherical inclusion. This extension opens the door for future application of this technique to various RVE types, facilitating efficient three-dimensional computational analyses at the macro-scale of such composite materials, significantly reducing computational time compared to FEM. The third innovation, facilitated by the integration of these surrogate models into a 3D Finite Element computational framework, lies in the assessment of these models scenarios encompassing intricate cases of extreme twisting and more importantly, buckling instabilities in thin-walled structures, thereby highlighting both the practical applicability and robustness of the proposed approach.},
keywords = {},
pubstate = {published},
tppubtype = {article}
}
2023

@article{Poya2023b,
title = {Variational schemes and mixed finite elements for large strain isotropic elasticity in principal stretches: Closed‐form tangent eigensystems, convexity conditions, and stabilised elasticity},
author = {Roman Poya and Rogelio Ortigosa and Antonio J. Gil},
doi = {10.1002/nme.7254},
issn = {1097-0207},
year = {2023},
date = {2023-08-30},
urldate = {2023-08-30},
journal = {Numerical Meth Engineering},
volume = {124},
number = {16},
pages = {3436--3493},
publisher = {Wiley},
abstract = {<jats:title>Abstract</jats:title><jats:p>A new computational framework for large strain elasticity in principal stretches is presented. Distinct from existing literature, the proposed formulation makes direct use of principal stretches rather than their squares that is, eigenvalues of Cauchy‐Green strain tensor. The proposed framework has three key features. First, the eigen‐decomposition of the tangent elasticity and initial (geometric) stiffness operators is obtained in closed‐form from principal information alone. Crucially, these newly found eigenvalues describe the general convexity conditions of isotropic hyperelastic energies. In other words, convexity is postulated concisely through tangent eigenvalues supplementing the original work of Ball (<jats:italic>Arch Ration Mech Anal</jats:italic>. 1976; 63(4): 337–403). Consequently, this novel finding opens the door for designing efficient automated Newton‐style algorithms with stabilised tangents via <jats:italic>closed‐form</jats:italic> semipositive definite projection or spectral shifting that converge irrespective of mesh resolution, quality, loading scenario and without relying on path‐following techniques. A critical study of closed‐form tangent stabilisation in the context of isotropic hyperelasticity is therefore undertaken in this work. Second, in addition to high order displacement‐based formulation, mixed Hu‐Washizu variational principles are formulated in terms of principal stretches by introducing stretch work conjugate Lagrange multipliers that enforce principal stretch‐stress compatibility. This is similar to enhanced strain methods. However, the resulting mixed finite element scheme is cost‐efficient, specially compared to approximating the entire strain tensors since the formulation is in the scalar space of singular values. Third, the proposed framework facilitates simulating rigid and stiff systems and those that are nearly‐inextensible in principal directions, a constituent of elasticity that cannot be easily studied using standard formulations.</jats:p>},
keywords = {},
pubstate = {published},
tppubtype = {article}
}

@article{Ortigosa-Martínez2023,
title = {Mathematical modeling, analysis and control in soft robotics: a survey},
author = {Rogelio Ortigosa and Jesús Martínez-Frutos and Carlos Mora-Corral and Pablo Pedregal and Francisco Periago},
doi = {10.1007/s40324-023-00334-4},
issn = {2281-7875},
year = {2023},
date = {2023-08-04},
urldate = {2023-08-04},
journal = {SeMA},
publisher = {Springer Science and Business Media LLC},
abstract = {<jats:title>Abstract</jats:title><jats:p>This paper reviews some recent advances in mathematical modeling, analysis and control, both from the theoretical and numerical viewpoints, in the emergent field of soft robotics. The presentation is not focused on specific prototypes of soft robots, but in a more general description of soft smart materials. The goal is to provide a unified and rigorous mathematical approach to open-loop control strategies for soft materials that hopefully might lay the seeds for future research in this field.</jats:p>},
keywords = {},
pubstate = {published},
tppubtype = {article}
}

@article{Poya2023,
title = {Geometric Optimisation Via Spectral Shifting},
author = {Roman Poya and Rogelio Ortigosa and Theodore Kim},
doi = {10.1145/3585003},
issn = {1557-7368},
year = {2023},
date = {2023-06-30},
urldate = {2023-06-30},
journal = {ACM Trans. Graph.},
volume = {42},
number = {3},
pages = {1--15},
publisher = {Association for Computing Machinery (ACM)},
abstract = {<jats:p>We present a geometric optimisation framework that can recover fold-over free maps from non-injective initial states using popular flip-preventing distortion energies. Since flip-preventing energies are infinite for folded configurations, we propose a new regularisation scheme that shifts the singular values of the deformation gradient. This allow us to re-use many existing algorithms, especially locally injective methods for initially folded maps. Our regularisation is suitable for both singular value- and invariant-based formulations, and systematically contributes multiple stabilisers to the Hessian. In contrast to proxy-based techniques, we maintain second-order convergence. Compact expressions for the energy eigensystems can be obtained for our extended stretch invariants, enabling the use of fast projected Newton solvers. Although spectral shifting in general has no theoretical guarantees that the global minimum is an injection, extensive experiments show that our framework is fast and extremely robust in practice, and capable of generating high-quality maps from severely distorted, degenerate and folded initialisations.</jats:p>},
keywords = {},
pubstate = {published},
tppubtype = {article}
}

@article{Franke2023,
title = {A novel mixed and energy‐momentum consistent framework for coupled nonlinear thermo‐electro‐elastodynamics},
author = {M. Franke and Felix Zähringer and Moritz Hille and Rogelio Ortigosa and P. Betsch and Antonio J. Gil},
doi = {10.1002/nme.7209},
issn = {1097-0207},
year = {2023},
date = {2023-05-30},
urldate = {2023-05-30},
journal = {Numerical Meth Engineering},
volume = {124},
number = {10},
pages = {2135--2170},
publisher = {Wiley},
abstract = {<jats:title>Abstract</jats:title><jats:p>A novel mixed framework and energy‐momentum consistent integration scheme in the field of coupled nonlinear thermo‐electro‐elastodynamics is proposed. The mixed environment is primarily based on a framework for elastodynamics in the case of polyconvex strain energy functions. For this elastodynamic framework, the properties of the so‐called tensor cross product are exploited to derive a mixed formulation via a Hu‐Washizu type extension of the strain energy function. Afterwards, a general path to incorporate nonpotential problems for mixed formulations is demonstrated. To this end, the strong form of the mixed framework is derived and supplemented with the energy balance as well as Maxwell's equations neglecting magnetic and time dependent effects. By additionally choosing an appropriate energy function, this procedure leads to a fully coupled thermo‐electro‐elastodynamic formulation which benefits from the properties of the underlying mixed framework. In addition, the proposed mixed framework facilitates the design of a new energy‐momentum consistent time integration scheme by employing discrete derivatives in the sense of Gonzalez. A one‐step integration scheme of second‐order accuracy is obtained which is shown to be stable even for large time steps. Eventually, the performance of the novel formulation is demonstrated in several numerical examples.</jats:p>},
keywords = {},
pubstate = {published},
tppubtype = {article}
}

@article{HORAK2023115695,
title = {A polyconvex transversely-isotropic invariant-based formulation for electro-mechanics: Stability, minimisers and computational implementation},
author = {Martin Horák and Antonio J. Gil and Rogelio Ortigosa and Martin Kružík},
url = {https://www.sciencedirect.com/science/article/pii/S0045782522006508},
doi = {https://doi.org/10.1016/j.cma.2022.115695},
issn = {0045-7825},
year = {2023},
date = {2023-01-01},
urldate = {2023-01-01},
journal = {Computer Methods in Applied Mechanics and Engineering},
volume = {403},
pages = {115695},
abstract = {The use of Electro-Active Polymers (EAPs) for the fabrication of evermore sophisticated miniaturised soft robotic actuators has seen an impressive development in recent years. The incorporation of crystallographic anisotropic micro-architectures, within an otherwise nearly uniform isotropic soft polymer matrix, has shown great potential in terms of advanced three-dimensional actuation (i.e. stretching, bending, twisting), especially at large strains, that is, beyond the onset of geometrical pull-in instabilities. From the computational point of view, the design of accurate and robust albeit efficient constitutive models is a very active area of research. This paper introduces a novel polyconvex phenomenological invariant-based transversely isotropic formulation (and relevant computational frameworks) for the simulation of transversely isotropic EAPs at large strains, where the ab initio satisfaction of polyconvexity is exploited to ensure the robustness of numerical results for any range of deformations and applied electric fields. The paper also presents key important results both in terms of the existence of minimisers and material stability of coupled electro-mechanics, enhancing previous works in the area of large strain elasticity. In addition, a comprehensive series of selected numerical examples is included in order to demonstrate the effect that the anisotropic orientation and the contrast of material properties, as well as the level of deformation and electric field, have upon the response of the EAP when subjected to large three-dimensional stretching, bending and torsion, including the possible development of wrinkling and the potential loss of ellipticity in ill-posed constitutive models.},
keywords = {},
pubstate = {published},
tppubtype = {article}
}

@article{ORTIGOSA2023346,
title = {Programming shape-morphing electroactive polymers through multi-material topology optimisation},
author = {Rogelio Ortigosa and Jesús Martínez-Frutos and Antonio J. Gil},
url = {https://www.sciencedirect.com/science/article/pii/S0307904X23000410},
doi = {https://doi.org/10.1016/j.apm.2023.01.041},
issn = {0307-904X},
year = {2023},
date = {2023-01-01},
urldate = {2023-01-01},
journal = {Applied Mathematical Modelling},
volume = {118},
pages = {346-369},
abstract = {This paper presents a novel engineering strategy for the design of Dielectric Elastomer (DE) based actuators, capable of attaining complex electrically induced shape morphing configurations. In this approach, a multilayered DE prototype, interleaved with compliant electrodes spreading across the entire faces of the DE, is considered. Careful combination of several DE materials, characterised by different material properties within each of the multiple layers of the device, is pursued. The resulting layout permits the generation of a heterogenous electric field within the device due to the spatial variation of the material properties within the layers and across them. An in-silico or computational approach has been developed in order to facilitate the design of new prototypes capable of displaying predefined electrically induced target configurations. Key features of this framework are: (i) use of a standard two-field Finite Element implementation of the underlying partial differential equations in reversible nonlinear electromechanics, where the unknown fields ot the resulting discrete problem are displacements and the scalar electric potential; (ii) introduction of a novel phase-field driven multi-material topology optimisation framework allowing for the consideration of several DE materials with different material properties, favouring the development of heterogeneous electric fields within the prototype. This novel multi-material framework permits, for the first time, the consideration of an arbitrary number of different N DE materials, by means of the introduction of N−1 phase-field functions, evolving independently over the different layers across the thickness of the device through N−1 Allen-Cahn type evolution equations per layer. A comprehensive series of numerical examples is analysed, with the aim of exploring the capability of the proposed methodology to propose efficient optimal designs. Specifically, the topology optimisation algorithm determines the topology of regions where different DE materials must be conveniently placed in order to attain complex electrically induced configurations.},
keywords = {},
pubstate = {published},
tppubtype = {article}
}
2022

@article{Remigio-Reyes2022,
title = {Topology optimization-driven design of added rib architecture system for enhanced free vibration response of thin-wall plastic components used in the automotive industry},
author = {Joel Omar Remigio-Reyes and Isaías E. Garduño and José Manuel Rojas-García and Hugo Arcos-Gutiérrez and Rogelio Ortigosa},
doi = {10.1007/s00170-022-10219-x},
issn = {1433-3015},
year = {2022},
date = {2022-11-00},
urldate = {2022-11-00},
journal = {Int J Adv Manuf Technol},
volume = {123},
number = {3-4},
pages = {1231--1247},
publisher = {Springer Science and Business Media LLC},
keywords = {},
pubstate = {published},
tppubtype = {article}
}

@article{ORTIGOSA2022115604,
title = {A computational framework for topology optimisation of flexoelectricity at finite strains considering a multi-field micromorphic approach},
author = {Rogelio Ortigosa and Jesús Martínez-Frutos and Antonio J. Gil},
url = {https://www.sciencedirect.com/science/article/pii/S0045782522005667},
doi = {https://doi.org/10.1016/j.cma.2022.115604},
issn = {0045-7825},
year = {2022},
date = {2022-01-01},
urldate = {2022-01-01},
journal = {Computer Methods in Applied Mechanics and Engineering},
volume = {401},
pages = {115604},
abstract = {This paper presents a novel in-silico framework for the design of flexoelectric energy harvesters at finite strains using topology optimisation. The main ingredients of this work can be summarised as follows: (i) a micromorphic continuum approach is exploited to account for size dependent effects in the context of finite strains, thus permitting the modelling and simulation of flexoelectric effects in highly deformable materials such as dielectric elastomers. A key feature of the multi-field (mixed) formulation pursued is its flexibility as it permits, upon suitable selection of material parameters, to degenerate into other families of high order gradient theories such as flexoelectric gradient elasticity. (ii) A novel energy interpolation scheme is put forward, whereby different interpolation strategies are proposed for the various contributions that the free energy density function is decomposed into. This has enabled to circumvent numerical artifacts associated with fictitious high flexoelectric effects observed in the vicinity of low and intermediate density regions, where extremely high strain gradients tend to develop. (iii) A weighted combination of efficiency-based measures and aggregation functions of the stress is proposed to remedy the shortcomings of state-of-the-art efficiency-based functionals, which promotes the development of hinges with unpractical highly localised large strain gradients. Finally, a series of numerical examples are analysed, studying the development of direct flexoelectricity induced by bending, compression and torsional deformations.},
keywords = {},
pubstate = {published},
tppubtype = {article}
}

@article{FRANKE2022114298,
title = {A thermodynamically consistent time integration scheme for non-linear thermo-electro-mechanics},
author = {M. Franke and Rogelio Ortigosa and Jesús Martínez-Frutos and Antonio J. Gil and P. Betsch},
url = {https://www.sciencedirect.com/science/article/pii/S0045782521005922},
doi = {https://doi.org/10.1016/j.cma.2021.114298},
issn = {0045-7825},
year = {2022},
date = {2022-01-01},
urldate = {2022-01-01},
journal = {Computer Methods in Applied Mechanics and Engineering},
volume = {389},
pages = {114298},
abstract = {The aim of this paper is the design of a new one-step implicit and thermodynamically consistent Energy–Momentum (EM) preserving time integration scheme for the simulation of thermo-electro-elastic processes undergoing large deformations. The time integration scheme takes advantage of the notion of polyconvexity and of a new tensor cross product algebra. These two ingredients are shown to be crucial for the design of so-called discrete derivatives fundamental for the calculation of the second Piola–Kirchhoff stress tensor, the entropy and the electric field. In particular, the exploitation of polyconvexity and the tensor cross product, enable the derivation of comparatively simple formulas for the discrete derivatives. This is in sharp contrast to much more elaborate discrete derivatives which are one of the main downsides of classical EM time integration schemes. The newly proposed scheme inherits the advantages of EM schemes recently published in the context of thermo-elasticity and electro-mechanics, whilst extending to the more generic case of nonlinear thermo-electro-mechanics. Furthermore, the manuscript delves into suitable convexity/concavity restrictions that thermo-electro-mechanical strain energy functions must comply with in order to yield physically and mathematically admissible solutions. Finally, a series of numerical examples will be presented in order to demonstrate robustness and numerical stability properties of the new EM scheme.},
keywords = {},
pubstate = {published},
tppubtype = {article}
}

@article{MARIN2022114358,
title = {Viscoelastic up-scaling rank-one effects in in-silico modelling of electro-active polymers},
author = {Francisco J. Marín and Rogelio Ortigosa and Jesús Martínez-Frutos and Antonio J. Gil},
url = {https://www.sciencedirect.com/science/article/pii/S0045782521006319},
doi = {https://doi.org/10.1016/j.cma.2021.114358},
issn = {0045-7825},
year = {2022},
date = {2022-01-01},
urldate = {2022-01-01},
journal = {Computer Methods in Applied Mechanics and Engineering},
volume = {389},
pages = {114358},
abstract = {This paper analyses the viscoelastic up-scaling effects in electro-active polymers endowed with a micro-structure architecture in the form of a rank-one laminate. The principles of rank-n homogenisation and thermodynamical consistency are combined in the context of extremely deformable dielectric elastomers actuated well beyond the onset of geometrical instabilities. To ensure the robustness of the resulting methodology, Convex Multi-Variable (CMV) energy density functionals enriched with a nonlinear continuum viscoelastic description are used to describe the physics of the individual microscopic constituents. The high nonlinearity of the visco-electro-mechanical problem is resolved via a monolithic multi-scale Newton–Raphson scheme with a Backward-Euler (implicit) time integration scheme. A tensor cross product operation between vectors and tensors and an additive decomposition of the micro-scale deformation gradient (in terms of macro-scale and fluctuation components) are used to considerably reduce the complexity of the algebra. The resulting computational framework permits to explore the time-dependent in-silico analysis of rank-one electro-active polymer composites exhibiting extremely complex deformation patterns, paying particular attention to viscoelastic up-scaling effects. A comprehensive series of numerical examples is presented, where specially revealing conclusions about the rate-dependency of the composite electro-active polymer are observed as a function of its microstructure orientation and viscoelastic content. In a rectangular film subjected to extreme bending deformation, two different deformation modes are observed with one prevailing mode depending on the laminate composition. For the case of a square membrane where extreme deformation induces buckling, it is shown that the viscoelastic contribution leads to larger values of (stable) deformation, due to the regularisation that viscoelasticity inherently provides.},
keywords = {},
pubstate = {published},
tppubtype = {article}
}

@article{ORTIGOSA2022141,
title = {Optimal control and design of magnetic field-responsive smart polymer composites},
author = {Rogelio Ortigosa and Jesús Martínez-Frutos and Carlos Mora-Corral and Pablo Pedregal and Francisco Periago},
url = {https://www.sciencedirect.com/science/article/pii/S0307904X21005096},
doi = {https://doi.org/10.1016/j.apm.2021.10.033},
issn = {0307-904X},
year = {2022},
date = {2022-01-01},
urldate = {2022-01-01},
journal = {Applied Mathematical Modelling},
volume = {103},
pages = {141-161},
abstract = {This paper presents a novel in-silico framework for the simultaneous optimal control and design of complex magnetic responsive polymer composite materials. State-of-the-art optimisation techniques are used in conjunction with the latest developments in the numerical solution of hard-magnetic soft materials undergoing large (potentially extreme) deformations, in order to address the challenging task of designing shape-morphing two-dimensional composite magnetic sheets. This paper introduces the following key novelties: (i) an optimisation-driven method for the simultaneous optimal control and design of the externally applied magnetic flux density as well as the remnant magnetisation of hard particles within the elastomer matrix, (ii) the well-posedness character of the optimisation problem is established by proving existence of solutions for both the underlying state equation and the control problem itself, (iii) a gradient-based optimisation algorithm is proposed for the numerical approximation of the problem, where explicit expressions of the continuous gradients are obtained by using the formal Lagrangian method. Furthermore, a series of numerical examples are presented in order to demonstrate the capability of the proposal as an alternative to intuition or experimentally-based approaches, representing an optimisation-driven method that facilitates the design of smart materials yielding complex magnetically induced shape morphing configurations.},
keywords = {},
pubstate = {published},
tppubtype = {article}
}

@article{KLEIN2022115501,
title = {Finite electro-elasticity with physics-augmented neural networks},
author = {Dominik K. Klein and Rogelio Ortigosa and Jesús Martínez-Frutos and Oliver Weeger},
url = {https://www.sciencedirect.com/science/article/pii/S004578252200514X},
doi = {https://doi.org/10.1016/j.cma.2022.115501},
issn = {0045-7825},
year = {2022},
date = {2022-01-01},
urldate = {2022-01-01},
journal = {Computer Methods in Applied Mechanics and Engineering},
volume = {400},
pages = {115501},
abstract = {In the present work, a machine learning based constitutive model for electro-mechanically coupled material behavior at finite deformations is proposed. Using different sets of invariants as inputs, an internal energy density is formulated as a convex neural network. In this way, the model fulfills the polyconvexity condition which ensures material stability, as well as thermodynamic consistency, objectivity, material symmetry, and growth conditions. Depending on the considered invariants, this physics-augmented machine learning model can either be applied for compressible or nearly incompressible material behavior, as well as for arbitrary material symmetry classes. The applicability and versatility of the approach is demonstrated by calibrating it on transversely isotropic data generated with an analytical potential, as well as for the effective constitutive modeling of an analytically homogenized, transversely isotropic rank-one laminate composite and a numerically homogenized cubic metamaterial. These examinations show the excellent generalization properties that physics-augmented neural networks offer also for multi-physical material modeling such as nonlinear electro-elasticity.},
keywords = {},
pubstate = {published},
tppubtype = {article}
}
2021

@article{Ortigosa2021c,
title = {Topology optimisation of stiffeners layout for shape-morphing of dielectric elastomers},
author = {Rogelio Ortigosa and Jesús Martínez-Frutos},
doi = {10.1007/s00158-021-03047-2},
issn = {1615-1488},
year = {2021},
date = {2021-12-00},
urldate = {2021-12-00},
journal = {Struct Multidisc Optim},
volume = {64},
number = {6},
pages = {3681--3703},
publisher = {Springer Science and Business Media LLC},
keywords = {},
pubstate = {published},
tppubtype = {article}
}

@article{Martínez-Frutos2021,
title = {Risk-averse approach for topology optimization of fail-safe structures using the level-set method},
author = {Jesús Martínez-Frutos and Rogelio Ortigosa},
doi = {10.1007/s00466-021-02058-6},
issn = {1432-0924},
year = {2021},
date = {2021-11-00},
urldate = {2021-11-00},
journal = {Comput Mech},
volume = {68},
number = {5},
pages = {1039--1061},
publisher = {Springer Science and Business Media LLC},
keywords = {},
pubstate = {published},
tppubtype = {article}
}

@article{Ortigosa2021b,
title = {Multi-resolution methods for the topology optimization of nonlinear electro-active polymers at large strains},
author = {Rogelio Ortigosa and Jesús Martínez-Frutos},
doi = {10.1007/s00466-021-02030-4},
issn = {1432-0924},
year = {2021},
date = {2021-08-00},
urldate = {2021-08-00},
journal = {Comput Mech},
volume = {68},
number = {2},
pages = {271--293},
publisher = {Springer Science and Business Media LLC},
keywords = {},
pubstate = {published},
tppubtype = {article}
}

@article{Ortigosa2021,
title = {Density-based topology optimisation considering nonlinear electromechanics},
author = {Rogelio Ortigosa and Jesús Martínez-Frutos and David Ruiz and Alberto Donoso and Jose C. Bellido},
doi = {10.1007/s00158-021-02886-3},
issn = {1615-1488},
year = {2021},
date = {2021-07-00},
urldate = {2021-07-00},
journal = {Struct Multidisc Optim},
volume = {64},
number = {1},
pages = {257--280},
publisher = {Springer Science and Business Media LLC},
keywords = {},
pubstate = {published},
tppubtype = {article}
}

@article{MARTINEZFRUTOS2021106677b,
title = {Robust topology optimization of continuum structures under uncertain partial collapses},
author = {Jesús Martínez-Frutos and Rogelio Ortigosa},
url = {https://www.sciencedirect.com/science/article/pii/S0045794921001991},
doi = {https://doi.org/10.1016/j.compstruc.2021.106677},
issn = {0045-7949},
year = {2021},
date = {2021-01-01},
urldate = {2021-01-01},
journal = {Computers & Structures},
volume = {257},
pages = {106677},
abstract = {This paper presents a novel probabilistic approach for fail-safe robust topology optimization with the following novelties: (1) the probability for failure to occur at a specified location is considered; (2) the possibility for random failure size is incorporated; (3) a multi-objective problem is pursued encompassing both the expected value of the structural performance and its variance as a robustness criterion. Compared against alternative worst-case-based formulations, the probabilistic framework employed allows designers to assume certain level of risk, avoiding undesirable increments in structural performance due to low probability damage configurations; (4) alternatively to most existing works within fail-safe topology optimization, considering density-based methods, this paper pursues for the first time an optimization technique where the structural boundary is represented implicitly by an iso-level of an optimality criterion field, which is gradually evolved using a bisection method. A key advantage of this technique is that it provides optimized solutions for different volume fractions during the optimization process, allowing to efficiently find a trade-off between structural performance, cost and robustness. Finally, numerical results are included demonstrating the ability of the proposed formulation to provide smooth and clearly defined structural boundaries and to enhance structural robustness with respect to conventional deterministic designs.},
keywords = {},
pubstate = {published},
tppubtype = {article}
}

@article{MARIN2021113567,
title = {A Convex Multi-Variable based computational framework for multilayered electro-active polymers},
author = {Francisco J. Marín and Jesús Martínez-Frutos and Rogelio Ortigosa and Antonio J. Gil},
url = {https://www.sciencedirect.com/science/article/pii/S0045782520307520},
doi = {https://doi.org/10.1016/j.cma.2020.113567},
issn = {0045-7825},
year = {2021},
date = {2021-01-01},
urldate = {2021-01-01},
journal = {Computer Methods in Applied Mechanics and Engineering},
volume = {374},
pages = {113567},
abstract = {This paper presents a novel computational framework for the in silico analysis of rank-one multilayered electro-active polymer composites exhibiting complex deformation patterns. The work applies the principles of rank-n homogenisation in the context of extremely deformable dielectric elastomers actuated beyond the onset of geometrical instabilities. Following previous work by the authors (Gil and Ortigosa, 2016; Ortigosa and Gil, 2016; Ortigosa and Gil, 2016) Convex Multi-Variable (CMV) energy density functionals are used to describe the physics of the individual microscopic constituents, which is shown to guarantee ab initio the existence of solutions for the microstructure problem, described in terms of the so-called deformation gradient and electric displacement amplitude vectors. The high nonlinearity of the quasi-static electro-mechanical problem is resolved via a monolithic multi-scale Newton–Raphson scheme, which is enhanced with a tailor-made arc length technique, used to circumvent the onset of geometrical instabilities. A tensor cross product operation between vectors and tensors and an additive decomposition of the micro-scale deformation gradient (in terms of macro-scale and fluctuation components) are used to considerably reduce the complexity of the algebra. The possible loss of ellipticity of the homogenised constitutive model is strictly monitored through the minors of the homogenised acoustic tensor. A series of numerical examples is presented in order to demonstrate the effect that the volume fraction, the contrast and the material properties, as well as the level of deformation and electric field, have upon the response of the composites when subjected to large three dimensional stretching, bending and torsion, including the possible development of wrinkling.},
keywords = {},
pubstate = {published},
tppubtype = {article}
}

@article{MARTINEZFRUTOS2021106677,
title = {Robust topology optimization of continuum structures under uncertain partial collapses},
author = {Jesús Martínez-Frutos and Rogelio Ortigosa},
url = {https://www.sciencedirect.com/science/article/pii/S0045794921001991},
doi = {https://doi.org/10.1016/j.compstruc.2021.106677},
issn = {0045-7949},
year = {2021},
date = {2021-01-01},
urldate = {2021-01-01},
journal = {Computers & Structures},
volume = {257},
pages = {106677},
abstract = {This paper presents a novel probabilistic approach for fail-safe robust topology optimization with the following novelties: (1) the probability for failure to occur at a specified location is considered; (2) the possibility for random failure size is incorporated; (3) a multi-objective problem is pursued encompassing both the expected value of the structural performance and its variance as a robustness criterion. Compared against alternative worst-case-based formulations, the probabilistic framework employed allows designers to assume certain level of risk, avoiding undesirable increments in structural performance due to low probability damage configurations; (4) alternatively to most existing works within fail-safe topology optimization, considering density-based methods, this paper pursues for the first time an optimization technique where the structural boundary is represented implicitly by an iso-level of an optimality criterion field, which is gradually evolved using a bisection method. A key advantage of this technique is that it provides optimized solutions for different volume fractions during the optimization process, allowing to efficiently find a trade-off between structural performance, cost and robustness. Finally, numerical results are included demonstrating the ability of the proposed formulation to provide smooth and clearly defined structural boundaries and to enhance structural robustness with respect to conventional deterministic designs.},
keywords = {},
pubstate = {published},
tppubtype = {article}
}

@article{doi:10.1137/19M1307299,
title = {Optimal Control of Soft Materials Using a Hausdorff Distance Functional},
author = {Rogelio Ortigosa and Jesús Martínez-Frutos and Carlos Mora-Corral and Pablo Pedregal and Francisco Periago},
url = {https://doi.org/10.1137/19M1307299},
doi = {10.1137/19M1307299},
year = {2021},
date = {2021-01-01},
urldate = {2021-01-01},
journal = {SIAM Journal on Control and Optimization},
volume = {59},
number = {1},
pages = {393-416},
abstract = {This paper addresses, from both theoretical and numerical standpoints, the problem of optimal control of hyperelastic materials characterized by means of polyconvex stored energy functionals. Specifically, inspired by Günnel and Herzog [Front. Appl. Math. Stat., 2 (2016)], a bio-inspired type of external action or control, which resembles the electro-activation mechanism of the human heart, is considered in this paper. The main contribution resides in the consideration of tracking-type cost functionals alternative to those generally used in this field, where the $L^2$ norm of the distance to a given target displacement field is the preferred option. Alternatively, the Hausdorff metric is, for the first time, explored in the context of optimal control in hyperelasticity. The existence of a solution for a regularized version of the optimal control problem is proved. A gradient-based method, which makes use of the concept of shape derivative, is proposed as a numerical resolution method. A series of numerical examples are included illustrating the viability and applicability of the Hausdorff metric in this new context. Furthermore, although not pursued in this paper, it must be emphasized that in contrast to $L^2$ norm tracking-cost functional types, the Hausdorff metric permits the use of potentially very different computational domains for both the target and the actuated soft continuum.},
keywords = {},
pubstate = {published},
tppubtype = {article}
}

@article{MARTINEZFRUTOS2021104594,
title = {In-silico design of electrode meso-architecture for shape morphing dielectric elastomers},
author = {Jesús Martínez-Frutos and Rogelio Ortigosa and Antonio J. Gil},
url = {https://www.sciencedirect.com/science/article/pii/S0022509621002386},
doi = {https://doi.org/10.1016/j.jmps.2021.104594},
issn = {0022-5096},
year = {2021},
date = {2021-01-01},
urldate = {2021-01-01},
journal = {Journal of the Mechanics and Physics of Solids},
volume = {157},
pages = {104594},
abstract = {This paper presents a novel in-silico tool for the design of complex multilayer Dielectric Elastomers (DEs) characterised by recently introduced layer-by-layer reconfigurable electrode meso-architectures. Inspired by cutting-edge experimental work at Clarke Lab (Harvard) Hajiesmaili and Clarke (2019), this contribution introduces a novel approach underpinned by a diffuse interface treatment of the electrodes, whereby a spatially varying electro-mechanical free energy density is introduced whose active properties are related to the electrode meso-architecture of choice. State-of-the-art phase-field optimisation techniques are used in conjunction with the latest developments in the numerical solution of electrically stimulated DEs undergoing large (potentially extreme) deformations, in order to address the challenging task of finding the most suitable electrode layer-by-layer meso-architecture that results in a specific three-dimensional actuation mode. The paper introduces three key novelties. First, the consideration of the phase-field method for the implicit definition of reconfigurable electrodes placed at user-defined interface regions. Second, the extension of the electrode in-surface phase-field functions to the surrounding dielectric elastomeric volume in order to account for the effect of the presence (or absence) of electrodes within the adjacent elastomeric layers. Moreover, an original energy interpolation scheme of the free energy density is put forward where only the electromechanical contribution is affected by the extended phase-field function, resulting in an equivalent spatially varying active material formulation. Third, consideration of a non-conservative Allen–Cahn type of law for the evolution of the in-surface electrode phase field functions, adapted to the current large strain highly nonlinear electromechanical setting. A series of proof-of-concept examples (in both circular and squared geometries) are presented in order to demonstrate the robustness of the methodology and its potential as a new tool for the design of new DE-inspired soft-robotics components. The ultimate objective is to help thrive the development of this technology through the in-silico production of voltage-tunable (negative and positive Gaussian curvature) DEs shapes beyond those obtained solely via trial-and-error experimental investigation.},
keywords = {},
pubstate = {published},
tppubtype = {article}
}
2020

@article{ORTIGOSA2020113395,
title = {A new energy–momentum time integration scheme for non-linear thermo-mechanics},
author = {Rogelio Ortigosa and Antonio J. Gil and Jesus Martínez-Frutos and M. Franke and Javier Bonet},
url = {https://www.sciencedirect.com/science/article/pii/S0045782520305806},
doi = {https://doi.org/10.1016/j.cma.2020.113395},
issn = {0045-7825},
year = {2020},
date = {2020-01-01},
urldate = {2020-01-01},
journal = {Computer Methods in Applied Mechanics and Engineering},
volume = {372},
pages = {113395},
abstract = {The aim of this paper is the design a new one-step implicit and thermodynamically consistent Energy–Momentum (EM) preserving time integration scheme for the simulation of thermo-elastic processes undergoing large deformations and temperature fields. Following Bonet et al. (2020), we consider well-posed constitutive models for the entire range of deformations and temperature. In that regard, the consideration of polyconvexity inspired constitutive models and a new tensor cross product algebra are shown to be crucial in order to derive the so-called discrete derivatives, fundamental for the construction of the algorithmic derived variables, namely the second Piola–Kirchoff stress tensor and the entropy (or the absolute temperature). The proposed scheme inherits the advantages of the EM scheme recently published by Franke et al. (2018), whilst resulting in a simpler scheme from the implementation standpoint. A series of numerical examples will be presented in order to demonstrate the robustness and applicability of the new EM scheme. Although the examples presented will make use of a temperature-based version of the EM scheme (using the Helmholtz free energy as the thermodynamical potential and the temperature as the thermodynamical state variable), we also include in an Appendix an entropy-based analogue EM scheme (using the internal energy as the thermodynamical potential and the entropy as the thermodynamical state variable).},
keywords = {},
pubstate = {published},
tppubtype = {article}
}

@article{MARTINEZFRUTOS2020888,
title = {Robust optimal control of stochastic hyperelastic materials},
author = {Jesus Martínez-Frutos and Rogelio Ortigosa and Pablo Pedregal and Francisco Periago},
url = {https://www.sciencedirect.com/science/article/pii/S0307904X20303772},
doi = {https://doi.org/10.1016/j.apm.2020.07.012},
issn = {0307-904X},
year = {2020},
date = {2020-01-01},
urldate = {2020-01-01},
journal = {Applied Mathematical Modelling},
volume = {88},
pages = {888-904},
abstract = {Soft robots are highly nonlinear systems made of deformable materials such as elastomers, fluids and other soft matter, that often exhibit intrinsic uncertainty in their elastic responses under large strains due to microstructural inhomogeneity. These sources of uncertainty might cause a change in the dynamics of the system leading to a significant degree of complexity in its controllability. This issue poses theoretical and numerical challenges in the emerging field of optimal control of stochastic hyperelasticity. This paper states and solves the robust averaged control in stochastic hyperelasticity where the underlying state system corresponds to the minimization of a stochastic polyconvex strain energy function. Two bio-inspired optimal control problems under material uncertainty are addressed. The expected value of the L2-norm to a given target configuration is minimized to reduce the sensitivity of the spatial configuration to variations in the material parameters. The existence of optimal solutions for the robust averaged control problem is proved. Then the problem is solved numerically by using a gradient-based method. Two numerical experiments illustrate both the performance of the proposed method to ensure the robustness of the system and the significant differences that may occur when uncertainty is incorporated in this type of control problems.},
keywords = {},
pubstate = {published},
tppubtype = {article}
}
2019

@article{Ortigosa2019,
title = {A new stabilisation approach for level-set based topology optimisation of hyperelastic materials},
author = {Rogelio Ortigosa and Jesús Martínez-Frutos and Antonio J. Gil
},
doi = {https://doi.org/10.1007/s00158-019-02324-5},
isbn = {1615-147X},
year = {2019},
date = {2019-02-01},
urldate = {2019-02-01},
journal = {Struct Multidisc Optim},
volume = {60},
pages = {2343–2371},
abstract = {This paper introduces a novel computational approach for level-set based topology optimisation of hyperelastic materials at large strains. This, to date, is considered an unresolved open problem in topology optimisation due to its extremely challenging nature. Two computational strategies have been proposed to address this problem. The first strategy resorts to an arc-length in the pre-buckling region of intermediate topology optimisation (TO) iterations where numerical difficulties arise (associated with nucleation, disconnected elements, etc.), and is then continued by a novel regularisation technique in the post-buckling region. In the second strategy, the regularisation technique is used for the entire loading process at each TO iteration. The success of both rests on the combination of three distinct key ingredients. First, the nonlinear equilibrium equations of motion are solved in a consistent incrementally linearised fashion by splitting the design load into a number of load increments. Second, the resulting linearised tangent elasticity tensor is stabilised (regularised) in order to prevent its loss of positive definiteness and, thus, avoid the loss of convexity of the discrete tangent operator. Third, and with the purpose of avoiding excessive numerical stabilisation, a scalar degradation function is applied on the regularised linearised elasticity tensor, based on a novel regularisation indicator field. The robustness and applicability of this new methodological approach are thoroughly demonstrated through an ample spectrum of challenging numerical examples, ranging from benchmark two-dimensional (plane stress) examples to larger scale three-dimensional applications. Crucially, the performance of all the designs has been tested at a post-processing stage without adding any source of artificial stiffness. Specifically, an arc-length Newton-Raphson method has been employed in conjunction with a ratio of the material parameters for void and solid regions of 10e-12.
},
keywords = {},
pubstate = {published},
tppubtype = {article}
}
2018

@article{Ortigosa2018b,
title = {An energy-momentum integration scheme based on a convex multi-variable framework for non-linear electro-elastodynamics},
author = {Rogelio Ortigosa and M. Franke and A. Janz and Antonio J. Gil and P. Betsch},
url = {https://www.sciencedirect.com/science/article/pii/S0045782518302007},
doi = {https://doi.org/10.1016/j.cma.2018.04.021},
issn = {0045-7825},
year = {2018},
date = {2018-09-01},
urldate = {2018-09-01},
journal = {Computer Methods in Applied Mechanics and Engineering},
volume = {339},
pages = {1-35},
abstract = {This paper introduces a new one-step second order accurate energy–momentum (EM) preserving time integrator for reversible electro-elastodynamics. The new scheme is shown to be extremely useful for the long-term simulation of electroactive polymers (EAPs) undergoing massive strains and/or electric fields. The paper presents the following main novelties. (1) The formulation of a new energy–momentumtime integrator scheme in the context of nonlinear electro-elastodynamics. (2) The consideration of well-posed ab initio convex multi-variable constitutive models. (3) Based on the use of alternative mixed variational principles, the paper introduces two different EM time integration strategies (one based on the Helmholtz’s and the other based on the internal energy). (4) The new time integrator relies on the definition of four discrete derivatives of the internal/Helmholtz energies representing the algorithmic counterparts of the work conjugates of the right Cauchy–Green deformation tensor, its co-factor, its determinant and the Lagrangian electric displacement field. (6) Proof of thermodynamic consistency and of second order accuracy with respect to time of the resulting algorithm is included. Finally, a series of numerical examples are included in order to demonstrate the robustness and conservation properties of the proposed scheme, specifically in the case of long-term simulations.},
keywords = {},
pubstate = {published},
tppubtype = {article}
}
@article{Garcia-Blanco2018,
title = {A polyconvex computational formulation for electro-activation in cardiac mechanics},
author = {Emilio Garcia-Blanco and Rogelio Ortigosa and Antonio J. Gil and C. H. Lee and Javier Bonet},
year = {2018},
date = {2018-07-01},
urldate = {2018-07-01},
journal = {Biomechanics and Modeling in Mechanobiology},
keywords = {},
pubstate = {published},
tppubtype = {article}
}

@article{Poya2018,
title = {On a family of numerical models for couple stress based exoelectricity for continua and beams},
author = {Roman Poya and Antonio J. Gil and Rogelio Ortigosa and Roberto Palma},
year = {2018},
date = {2018-07-01},
urldate = {2018-07-01},
journal = {Journal of the Mechanics and Physics of Solids},
keywords = {},
pubstate = {published},
tppubtype = {article}
}

@article{Poya2018b,
title = {A curvilinear high order finite element framework for electro-mechanics: From linearised electro-elasticity to massively deformable dielectric elastomers},
author = {Roman Poya and Antonio J. Gil and Rogelio Ortigosa},
url = {https://www.sciencedirect.com/science/article/pii/S0045782517306503},
doi = {https://doi.org/10.1016/j.cma.2017.09.020},
year = {2018},
date = {2018-02-01},
urldate = {2018-02-01},
journal = {Computer Methods in Applied Mechanics and Engineering},
volume = {329},
pages = {75-117},
abstract = {This paper presents a high order finite element implementation of the convex multi-variable electro-elasticity for large deformations large electric fields analyses and its particularisation to the case of small strains through a staggered scheme. With an emphasis on accurate geometrical representation, a high performance curvilinear finite element framework based on an a posteriori mesh deformation technique is developed to accurately discretise the underlying displacement-potential variational formulation. The performance of the method under near incompressibility and bending actuation scenarios is analysed with extremely thin and highly stretched components and compared to the performance of mixed variational principles recently reported by Gil and Ortigosa (2016) and Ortigosa and Gil (2016). Although convex multi-variable constitutive models are elliptic hence, materially stable for the entire range of deformations and electric fields, other forms of physical instabilities are not precluded in these models. In particular, physical instabilities present in dielectric elastomers such as pull-in instability, snap-through and the formation, propagation and nucleation of wrinkles and folds are numerically studied with a detailed precision in this paper, verifying experimental findings. For the case of small strains, the essence of the approach taken lies in guaranteeing the objectivity of the resulting work conjugates, by starting from the underlying convex multi-variable internal energy, whence avoiding the need for further symmetrisation of the resulting Maxwell and Minkowski-type stresses at small strain regime. In this context, the nonlinearity with respect to electrostatic counterparts such as electric displacements is still retained, hence resulting in a formulation similar but more competitive with the existing linearised electro-elasticity approaches. Virtual prototyping of many application-oriented dielectric elastomers are carried out with an eye on pattern forming in soft robotics and other potential medical applications.},
keywords = {},
pubstate = {published},
tppubtype = {article}
}
2017

@article{Poya2017,
title = {A high performance data parallel tensor contraction framework: Application to coupled electro-mechanics},
author = {Roman Poya and Rogelio Ortigosa and Antonio J. Gil},
url = {https://www.sciencedirect.com/science/article/pii/S0010465517300681},
doi = {https://doi.org/10.1016/j.cpc.2017.02.016},
issn = {0010-4655},
year = {2017},
date = {2017-07-01},
urldate = {2017-07-01},
journal = {Computer Physics Communications},
volume = {216},
pages = {35-52},
abstract = {The paper presents aspects of implementation of a new high performance tensor contraction framework for the numerical analysis of coupled and multi-physics problems on streaming architectures. In addition to explicit SIMD instructions and smart expression templates, the framework introduces domain specific constructs for the tensor cross product and its associated algebra recently rediscovered by Bonet et al. (2015, 2016) in the context of solid mechanics. The two key ingredients of the presented expression template engine are as follows. First, the capability to mathematically transform complex chains of operations to simpler equivalent expressions, while potentially avoiding routes with higher levels of computational complexity and, second, to perform a compile time depth-first or breadth-first search to find the optimal contraction indices of a large tensor network in order to minimise the number of floating point operations. For optimisations of tensor contraction such as loop transformation, loop fusion and data locality optimisations, the framework relies heavily on compile time technologies rather than source-to-source translation or JIT techniques. Every aspect of the framework is examined through relevant performance benchmarks, including the impact of data parallelism on the performance of isomorphic and nonisomorphic tensor products, the FLOP and memory I/O optimality in the evaluation of tensor networks, the compilation cost and memory footprint of the framework and the performance of tensor cross product kernels. The framework is then applied to finite element analysis of coupled electro-mechanical problems to assess the speed-ups achieved in kernel-based numerical integration of complex electroelastic energy functionals. In this context, domain-aware expression templates combined with SIMD instructions are shown to provide a significant speed-up over the classical low-level style programming techniques.},
keywords = {},
pubstate = {published},
tppubtype = {article}
}

@article{andAntonioGil2017,
title = {A framework for polyconvex large strain phase-field methods to fracture},
author = {C. Hesch and Antonio J. Gil and Rogelio Ortigosa and M. Dittmann and C. Bilgen and P. Betsch and M. Franke and A. Janz and K.Weinberg},
url = {https://www.sciencedirect.com/science/article/pii/S0045782516309677},
doi = {https://doi.org/10.1016/j.cma.2016.12.035},
issn = {0045-7825},
year = {2017},
date = {2017-04-15},
urldate = {2017-04-15},
journal = {Computer Methods in Applied Mechanics and Engineering},
volume = {317},
pages = {649-683},
abstract = {Variationally consistent phase-field methods have been shown to be able to predict complex three-dimensional crack patterns. However, current computational methodologies in the context of large deformations lack the necessary numerical stability to ensure robustness in different loading scenarios. In this work, we present a novel formulation for finite strain polyconvex elasticity by introducing a new anisotropic split based on the principal invariants of the right Cauchy–Green tensor, which always ensures polyconvexity of the resulting strain energy function. The presented phase-field approach is embedded in a sophisticated isogeometrical framework with hierarchical refinement for three-dimensional problems using a fourth order Cahn–Hilliard crack density functional with higher-order convergence rates for fracture problems. Additionally, we introduce for the first time a Hu–Washizu mixed variational formulation in the context of phase-field problems, which permits the novel introduction of a variationally consistent stress-driven split. The new polyconvex phase-field fracture formulation guarantees numerical stability for the full range of deformations and for arbitrary hyperelastic materials.},
keywords = {},
pubstate = {published},
tppubtype = {article}
}
2016

@article{Ortigosa2016b,
title = {A computational framework for large strain nearly and truly incompressible electromechanics based on convex multi-variable strain energies},
author = {Rogelio Ortigosa and Antonio J. Gil and C. H. Lee},
url = {https://www.sciencedirect.com/science/article/pii/S0045782516302286},
doi = {https://doi.org/10.1016/j.cma.2016.06.025},
issn = {0045-7825},
year = {2016},
date = {2016-10-01},
urldate = {2016-10-01},
journal = {Computer Methods in Applied Mechanics and Engineering},
volume = {310},
pages = {297-334},
abstract = {The series of papers published by Gil and Ortigosa (Gil and Ortigosa, 2016; Ortigosa and Gil, 2016, 0000) introduced a new convex multi-variable variational and computational framework for the numerical simulation of Electro Active Polymers (EAPs) in scenarios characterised by extreme deformations and/or extreme electric fields. Building upon this body of work, five key novelties are incorporated in this paper. First, a generalisation of the concept of multi-variable convexity to energy functionals additively decomposed into isochoric and volumetric components. This decomposition is typical of nearly and truly incompressible materials, group which represents the majority of the most relevant EAPs. Second, convexification or regularisation strategies are applied to a priori non-convex multi-variable isochoric functionals to yield physically meaningful convex multi-variable functionals. Third, based on the mixed variational principles introduced in Gil and Ortigosa (2016) in the context of compressible electro-elasticity, a novel extended Hu–Washizu mixed variational principle for nearly and truly incompressible scenarios is presented. From the computational standpoint, a static condensation procedure is applied in order to condense out the element-wise extra fields, the resulting formulation having a comparable cost to the more standard three-field displacement-potential-pressure mixed formulation. Fourth, the computational framework for the three-field mixed variational principle in nearly and truly incompressible scenarios is also presented. In this case, the novelty resides in the consideration of convex multi-variable energy functionals. Ultimately, this leads to the definition of new tangent operators for the Helmholtz’s energy functional in the specific context of incompressible electro-elasticity. Fifth, a Petrov–Galerkin stabilisation technique is applied on the three-field formulation for the circumvention of the Ladyz˘enskaja–Babus˘ka–Brezzi (LBB) condition, enabling the use of linear tetrahedral finite elements for the interpolation of the unknowns of the problem. Finally, a series of challenging numerical examples is presented in order to provide an exhaustive comparison of the different variational formulations presented in this paper.},
keywords = {},
pubstate = {published},
tppubtype = {article}
}

@article{Ortigosa2016c,
title = {A new framework for large strain electromechanics based on convex multi-variable strain energies: Conservation laws, hyperbolicity and extension to electro-magneto- mechanics},
author = {Rogelio Ortigosa and Antonio J. Gil},
url = {https://www.sciencedirect.com/science/article/pii/S0045782516303991},
doi = {https://doi.org/10.1016/j.cma.2016.05.019},
issn = {0045-7825},
year = {2016},
date = {2016-09-01},
urldate = {2016-09-01},
journal = {Computer Methods in Applied Mechanics and Engineering,},
volume = {309},
pages = {202-242},
abstract = {This work is the third on a series of papers by Gil and Ortigosa (Gil and Ortigosa 2016; Ortigosa and Gil 2016) on the development of a new computational framework for the analysis of Electro Active Polymers, where the concept of polyconvexity (Ball 1976) is extended to the case of electro-magneto-mechanical energy functionals. Specifically, four key novelties are incorporated in this paper. Firstly, a new set of first order hyperbolic equations is presented in the context of nonlinear electro-magneto-elasticity, including conservation laws for all the fields of the extended set of arguments which determine the convex multi-variable nature of the internal energy. Secondly, the one-to-one and invertible relationship between this extended set and its associated entropy conjugate set enables the definition of a generalised convex entropy function, resulting in the symmetrisation of the system when expressed in terms of the entropy variables. Thirdly, this paper shows that, after careful analysis of the eigenvalue structure of the system, the definition of multi-variable convexity in Gil and Ortigosa (2016) leads to positive definiteness of the electro-magneto-acoustic tensor. Therefore, multi-variable convexity ensures the satisfaction of the Legendre–Hadamard condition, hence showing that the speeds of propagation of acoustic and electro-magnetic waves in the neighbourhood of a stationary point are real. Finally, under a characteristic experimental set-up for electrostrictive dielectric elastomers, a study of the material stability of convex and non-convex multi-variable constitutive models is carried out.},
keywords = {},
pubstate = {published},
tppubtype = {article}
}

@article{Bonet2016,
title = {On a tensor cross product based formulation of large strain solid mechanics},
author = {Javier Bonet and Antonio J. Gil and Rogelio Ortigosa},
url = {https://www.sciencedirect.com/science/article/pii/S0045782516303991},
doi = {https://doi.org/10.1016/j.ijsolstr.2015.12.030},
issn = {0020-7683},
year = {2016},
date = {2016-05-01},
urldate = {2016-05-01},
journal = {International Journal of Solids and Structures},
volume = {84},
pages = {49-63},
abstract = {This paper describes in detail the formulation of large strain solid mechanics based on the tensor cross product, originally presented by R. de Boer (1982) and recently re-introduced by Bonet et al. (2015a) and Bonet et al. (2015b). The paper shows how the tensor cross product facilitates the algebra associated with the area and volume maps between reference and final configurations. These maps, together with the fibre map, make up the fundamental kinematic variables in polyconvex elasticity. The algebra proposed leads to novel expressions for the tangent elastic operator which neatly separates material from geometrical dependencies. The paper derives new formulas for the spatial and material stress and their corresponding elasticity tensors. These are applied to the simple case of a Mooney–Rivlin material model. The extension to transversely isotropic material models is also considered.},
keywords = {},
pubstate = {published},
tppubtype = {article}
}

@article{Ortigosa2016e,
title = {A new framework for large strain electromechanics based on convex multi- variable strain energies: Finite Element discretisation and computational implementation},
author = {Rogelio Ortigosa and Antonio J. Gil},
url = {https://www.sciencedirect.com/science/article/pii/S0045782515004090},
doi = {https://doi.org/10.1016/j.cma.2015.12.007},
issn = {0045-7825},
year = {2016},
date = {2016-04-15},
urldate = {2016-04-15},
journal = {Computer Methods in Applied Mechanics and Engineering},
volume = {302},
pages = {329-360},
abstract = {In Gil and Ortigosa (2016), Gil and Ortigosa introduced a new convex multi-variable framework for the numerical simulation of Electro Active Polymers (EAPs) in the presence of extreme deformations and electric fields. This extends the concept of polyconvexity to strain energies which depend on non-strain based variables. The consideration of the new concept of multi-variable convexity guarantees the well posedness of generalised Gibbs’ energy density functionals and, hence, opens up the possibility of a new family of mixed variational principles. The aim of this paper is to present, as an example, the Finite Element implementation of two of these mixed variational principles. These types of enhanced methodologies are known to be necessary in scenarios in which the simpler displacement-potential based formulation yields non-physical results, such as volumetric locking, bending and shear locking, pressure oscillations and electro-mechanical locking, to name but a few. Crucially, the use of interpolation spaces in which some of the unknown fields are described as piecewise discontinuous across elements can be used in order to efficiently condense these fields out. This results in mixed formulations with a computational cost comparable to that of the displacement-potential based approach, yet far more accurate. Finally, a series of very challenging numerical examples are presented in order to demonstrate the accuracy, robustness and efficiency of the proposed methodology.},
keywords = {},
pubstate = {published},
tppubtype = {article}
}

@article{Ortigosa2016d,
title = {A new framework for large strain electromechanics based on convex multi- variable strain energies: variational formulation and material characterisation},
author = {Rogelio Ortigosa and Antonio J. Gil},
url = {https://www.sciencedirect.com/science/article/pii/S0045782515004168},
doi = {https://doi.org/10.1016/j.cma.2015.11.036},
year = {2016},
date = {2016-04-15},
urldate = {2016-04-15},
journal = {Computer Methods in Applied Mechanics and Engineering},
volume = {302},
pages = {293-328},
abstract = {Following the recent work of Bonet et al. (2015), this paper postulates a new convex multi-variable variational framework for the analysis of Electro Active Polymers (EAPs) in the context of reversible nonlinear electro-elasticity. This extends the concept of polyconvexity (Ball, 1976) to strain energies which depend on non-strain based variables introducing other physical measures such as the electric displacement. Six key novelties are incorporated in this work. First, a new definition of the electro-mechanical internal energy is introduced expressed as a convex multi-variable function of a new extended set of electromechanical arguments. Crucially, this new definition of the internal energy enables the most accepted constitutive inequality, namely ellipticity, to be extended to the entire range of deformations and electric fields and, in addition, to incorporate the electro-mechanical energy of the vacuum, and hence that for ideal dielectric elastomers, as a degenerate case. Second, a new extended set of variables, work conjugate to those characterising the new definition of multi-variable convexity, is introduced in this paper. Third, both new sets of variables enable the definition of novel extended Hu–Washizu type of mixed variational principles which are presented in this paper for the first time in the context of nonlinear electro-elasticity. Fourth, some simple strategies to create appropriate convex multi-variable energy functionals (in terms of convex multi-variable invariants) by incorporating minor modifications to a priori non-convex multi-variable functionals are also presented. Fifth, a tensor cross product operation (de Boer, 1982) used in Bonet et al. (2015) to facilitate the algebra associated with the adjoint of the deformation gradient tensor is incorporated in the proposed variational electro-mechanical framework, leading to insightful representations of otherwise complex algebraic expressions. Finally, under a characteristic experimental setup in dielectric elastomers, the behaviour of a convex multi-variable constitutive model capturing some intrinsic nonlinear effects such as electrostriction, is numerically studied.},
keywords = {},
pubstate = {published},
tppubtype = {article}
}

@article{Gil2016,
title = {A first order hyperbolic framework for large strain computational solid dynamics. Part II: Total Lagrangian compressible, nearly incompressible and truly incompressible elasticity},
author = {Antonio J. Gil and C. H. Lee and Javier Bonet and Rogelio Ortigosa},
url = {https://www.sciencedirect.com/science/article/pii/S0045782515003631},
doi = {https://doi.org/10.1016/j.cma.2015.11.010},
issn = {0045-7825},
year = {2016},
date = {2016-03-01},
urldate = {2016-03-01},
journal = {Computer Methods in Applied Mechanics and Engineering},
volume = {300},
pages = {146-181},
abstract = {In Part I of this series, Bonet et al. (2015) introduced a new computational framework for the analysis of large strain isothermal fast solid dynamics, where a mixed set of Total Lagrangian conservation laws was presented in terms of the linear momentum and an extended set of strain measures, namely the deformation gradient, its co-factor and its Jacobian. The main aim of this paper is to expand this formulation to the case of nearly incompressible and truly incompressible materials. The paper is further enhanced with three key novelties. First, the use of polyconvex nearly incompressible strain energy functionals enables the definition of generalised convex entropy functions and associated entropy fluxes. Two variants of the same formulation can then be obtained, namely, conservation-based and entropy-based, depending on the unknowns of the system. Crucially, the study of the eigenvalue structure of the system is carried out in order to demonstrate its hyperbolicity and, thus, obtain the correct time step bounds for explicit time integrators. Second, the development of a stabilised Petrov–Galerkin framework is presented for both systems of hyperbolic equations, that is, when expressed in terms of either conservation or entropy variables. Third, an adapted fractional step method, built upon the work presented in Gil et al. (2014), is presented to extend the range of applications towards the incompressibility limit. Finally, a series of numerical examples are presented in order to assess the applicability and robustness of the proposed formulation. The overall scheme shows excellent behaviour in compressible, nearly incompressible and truly incompressible scenarios, yielding equal order of convergence for velocities and stresses.},
keywords = {},
pubstate = {published},
tppubtype = {article}
}

@article{Ortigosa2016f,
title = {A computational framework for polyconvex large strain elasticity for geometrically exact beam theory},
author = {Rogelio Ortigosa and Antonio J. Gil and Javier Bonet and Christian Hesch},
url = {https://link.springer.com/article/10.1007/s00466-015-1231-5},
doi = {https://doi.org/10.1007/s00466-015-1231-5},
issn = {0178-7675},
year = {2016},
date = {2016-02-01},
urldate = {2016-02-01},
journal = {Computational Mechanics},
volume = {57},
pages = {277–303},
abstract = {In this paper, a new computational framework is presented for the analysis of nonlinear beam finite elements subjected to large strains. Specifically, the methodology recently introduced in Bonet et al. (Comput Methods Appl Mech Eng 283:1061–1094, 2015) in the context of three dimensional polyconvex elasticity is extended to the geometrically exact beam model of Simo (Comput Methods Appl Mech Eng 49:55–70, 1985), the starting point of so many other finite element beam type formulations. This new variational framework can be viewed as a continuum degenerate formulation which, moreover, is enhanced by three key novelties. First, in order to facilitate the implementation of the sophisticated polyconvex constitutive laws particularly associated with beams undergoing large strains, a novel tensor cross product algebra by Bonet et al. (Comput Methods Appl Mech Eng 283:1061–1094, 2015) is adopted, leading to an elegant and physically meaningful representation of an otherwise complex computational framework. Second, the paper shows how the novel algebra facilitates the re-expression of any invariant of the deformation gradient, its cofactor and its determinant in terms of the classical beam strain measures. The latter being very useful whenever a classical beam implementation is preferred. This is particularised for the case of a Mooney–Rivlin model although the technique can be straightforwardly generalised to other more complex isotropic and anisotropic polyconvex models. Third, the connection between the two most accepted restrictions for the definition of constitutive models in three dimensional elasticity and beams is shown, bridging the gap between the continuum and its degenerate beam description. This is carried out via a novel insightful representation of the tangent operator.},
keywords = {},
pubstate = {published},
tppubtype = {article}
}